Additive strategies: Video and teaching guide

Use this video to explore the use of computation strategies, rounding and estimation in real-world, additive situations.

The video builds on number sense and place value with a focus on presenting realistic problems that invite additive thinking. A range of strategies such as compensation and partitioning are demonstrated. Estimation and rounding are highlighted before mental computation strategies are used to find a precise answer.

The problems presented target students in Years 2, 3 and 4. However, the video can be used with students at higher levels to review and explore applications of estimation, rounding and mental computation strategies in everyday scenarios. While similar style problems can be used, substitute larger numbers to increase complexity.

Australian Curriculum V9 Mathematics
Years 2–4: Number
AC9M2N02, AC9M3N03, AC9M4N06, AC9M4N07

Numeracy Progression
Additive strategies: P8
Number and place value: P7

*P stands for Progression level

Watch the video below with your students (full screen recommended).

Video duration: 4 min 29 sec

Suggestions to follow up video

Objective: To recognise and use landmark numbers where useful when solving addition and subtraction problems.

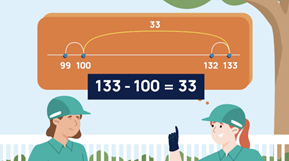
The first situation in the video asks the characters to work out the difference between 99 and 132. While Georgina’s solution path is different to Rose’s, both make use of 100 as a landmark number to make the calculation easier.

Two children, with child one explaining her maths thinking.Two children, with child two explaining her maths thinking.

Two children, where child number one is explaining her maths thinking to work out the difference between 99 and 132. A number line shows adding 1 to 99 to make 100, then adding 32 to make 132. Her answer is in a box: 1 plus 32 equals 33.
Two children, where child number two is explaining her maths thinking working out the difference between 99 and 132. A number line shows adding 1 to 99 to make 100, then adding 1 to 132 keeping the difference the same. It shows a difference of 33. Her answer is in a box: 133 minus 100 equals 33.

In the video, landmark numbers are defined as any number that is easier to think about or work with.

Three number lines showing landmark numbers.

Three number lines showing landmark numbers. The first number line shows 97 is close to the landmark number of 100. A second number line shows 96 is close to the landmark number of 100. The third number line shows 47 is close to the landmark number of 50.

Role of teacher: Help students see numbers such as multiples of tens and multiples of a hundred as landmark numbers, and that these numbers can be useful as anchoring points when solving addition and subtraction problems. Be sure to keep an open mind with regard to what constitutes a landmark number for individuals.

Objective: To recognise instances where the ‘keeping a constant difference’ strategy is useful. Use the strategy to help solve subtraction problems and recognise the role of landmark numbers.

The first situation in the video requires the characters to work out the difference between 99 and 132.  Rose’s solution path (the second solution path) involves ‘keeping a constant difference’. She rounds 99 up to 100 (a landmark number) and, in order to keep a constant difference, also rounds 132 up to 133.

Two children, with child number one explaining her maths thinking.

Two children, with child number one explaining her maths thinking working out the difference between 99 and 132. A number line shows adding 1 to 99 to make 100, then adding 1 to 132 keeping the difference the same. It shows a difference of 33. Her answer is in a box: 133 subtract 100 equals 33.

Role of teacher: Help students see how the ‘keeping a constant difference’ strategy maintains the same distance or quantity between the two numbers. It’s a strategy that often involves the use of landmark numbers (see previous activity).

 

Objective: Apply and evaluate different computation strategies in contexts involving money.

The second situation in the video requires Ali to determine whether he has enough cash to purchase both a t-shirt ($29) and  jeans ($117). Ali shares his mental process which involves the use of rounding to estimate the total cost. He then does a mental calculation that models transformation of addends, partitioning, and use of a number line. It’s figuring out the precise answer that determines whether or not he can make the purchase.

Role of teacher: Help students to see that many solution paths are made up of specific strategies. Use this task to build student understanding and vocabulary around different strategies used with the task of paying close attention to Ali’s solution path shown in the animation.

Download lesson 

Objective: To know that there are multiple ways to solve a problem efficiently and that different people can have a different preferred strategy when solving a problem. Be able to evaluate solution paths and determine whether they make sense for solving that particular problem.

The video explores a range of additive strategies to make calculations in everyday situations. It demonstrates that individuals are able to generate their own strategies, using what they know about numbers, to solve problems.

Role of teacher: Encourage students to think about an efficient strategy as a strategy that (1) makes sense to the person using it and (2) provides clear evidence of precision. In other words, there is more than one way to solve a problem efficiently.

Help students see that, depending on the prior knowledge and experience of each person, what counts as ‘most efficient’ at any moment for one person is likely to differ in another. What is ‘most efficient’ for each person will also evolve over time as they discover and develop new strategies.