Planning tool

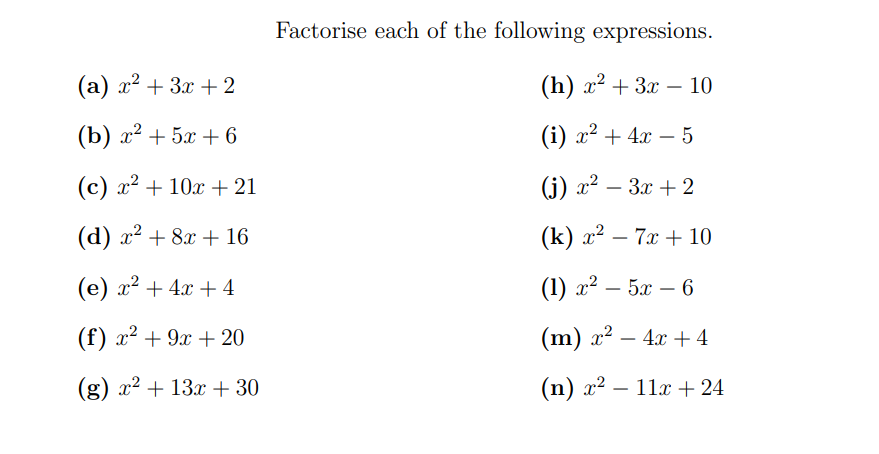
Students:

• expand, factorise and simplify quadratic expressions with integer coefficients
• convert between expanded and completed square forms of quadratic expressions
• relate quadratic expressions to diagrams and corresponding algebraic methods
• simplify exponential expressions involving combinations of products, quotients and powers of numbers and variables with
  integer exponents
• solve simple equations involving exponential expressions in one variable that have an integer solution.

Some students may:

• not correctly apply the distributive property when expanding the product of two binomial expressions, such as simply missing out middle terms, incorrectly thinking that (x + 3)² = x² + 9 or that (2x – 4)² = 4x² – 16.
• multiply rather than add exponents when simplifying exponential expressions involving multiplication.
• divide, rather than subtract exponents when simplifying exponential expressions involving division.
• confuse the power of an exponential expression with the product of two exponential expressions, such as thinking (3⁴)² = 3⁴ × 3² rather than 3⁸.
• not realise that a pronumeral without the exponent showing always has an exponent of 1 (for example, 2 = 2¹, x = x¹) or that base integers with an exponent of 0 equals 1 (3⁰ = 1, x⁰ = 1).
• not realise that a negative exponent corresponds to the reciprocal of the corresponding positive exponent.

The Learning from home activities are designed to be used flexibly by teachers, parents and carers, as well as the students themselves. They can be used in a number of ways including to consolidate and extend learning done at school or for home schooling.

Learning intentions

• We are learning to consolidate expanding the product of two binomial expressions, (ax + b)(cx + d).
• We are learning to consolidate factorising non-monic quadratic expressions.

Why are we learning about this?

Quadratic functions and their rules can be used to model situations and solve problems involving curves, areas, patterns and movements. 

Formulating and manipulating quadratic expressions where the coefficient of x² is different from 1 covers the full range of quadratic functions.

What to do

Practise

Use an interactive problem solver or graphics calculator to practise expanding quadratic expressions such as (2x – 3)(4x + 5):

• Complete the problem by hand on paper.
• Check your solution by typing the expression (2x – 3)(4x + 5) into the digital tool.  
• If your tool allows, view the solution steps to see if your working is correct.

Now test yourself!

Expand each of the following and check your solution.

1. (4x + 3)(5x + 5)
2. (8x + 2)(7x – 2)
3. (5x – 6)(7x + 4)
4. (9x – 4)(3x – 5)

Try this

A group of students was asked to expand (3x + 4)(2x – 5) and between them they obtained four different answers, listed below.

Identify the three incorrect answers and explain the common error made in each case:

1. 6x² – 20
2. 6x² – 7x – 20
3. 6x² + 8x – 20
4. 6x² + 23x – 20

Practise 

• Use a digital problem solver or graphics calculator to practise factorising quadratic expressions such as 3x² – 10x + 8
• Complete the problem by hand on paper.
• Check your solution by typing the expression 3x² – 10x + 8 into the digital tool.  
• If your tool allows, view the solution steps to see if your working is correct.

You try!

Factorise each of the following and check your solution:

1. 2x² + 11x + 5
2. 6x² + 10x – 4
3. 8x² – 20x + 12
4. 10x² – 11x – 6

Success criteria – quadratic expressions

• I can expand the product of two binomial expressions.
• I can factorise quadratic expressions where the coefficient of x² is different from 1.