Planning tool

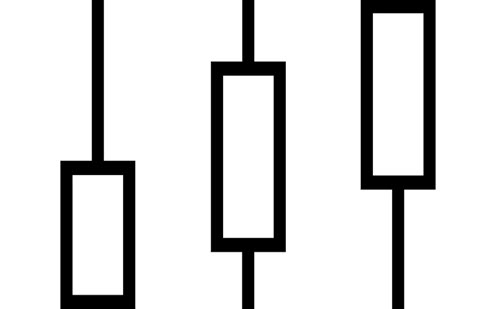
Students:

• compare data distributions for continuous numerical variables using comprehensive mathematical vocabulary
• construct box plots and can analyse shape, spread, outliers and the centre for a given context and data-set
• compare box plots side by side and draw reasonable conclusions about the datasets
• construct scatterplots noting the strength of relationship between two variables (direction, linearity, strength)
• construct two-way tables and make connections between trends or relationships between categorical variables.

Some students may:

• be adept at learning and constructing box plots, scatterplots and two-way tables, but not fully comprehend why these are used to represent data.
• have never seen these graphs in real-world contexts and will need to be exposed to relevant box plots (for example, using academic data).
• require a refresher in key vocabulary.
• be confused with percentiles and how they are represented by each marker on the box plot.
• work easily with numbers and categories but fail to understand them in a real-world context.
• confuse types of data and the appropriate forms of data display.
• find it difficult to make conclusions that are grounded in data, rather than hypotheses.

The Learning from home activities are designed to be used flexibly by teachers, parents and carers, as well as the students themselves. They can be used in a number of ways including to consolidate and extend learning done at school or for home schooling.

Learning intention

• We are learning to generate box plots using digital technology.
• We will compare the centre, spread, shape and presence of outliers in the distributions.

Why are we learning about this?

You are growing up in a world that is fuelled by data. Many industries now expect their workers to be data literate and to make evidence-based decisions. Your teachers are prime examples. Behind the scenes, they are analysing data generated by your academic journey to provide the best and most consistent learning experience for each cohort and individual.

What to do

Step into the shoes of your teachers for a day and see if you can compare sets of fictional mathematics test scores!

1. You are going to use spreadsheet software, like Excel, to create fictional sets of test scores. You may wish to model this on your current year level or you can make your test scores up and enter them manually. I am going to teach you how to do it automatically.
2. Open up a new spreadsheet and use Row 1 to name each class with sensible titles: Class 1, Class 2, Class 3 etc. See the table below.
3. The function '=randbetween(1,100)' will generate random class test scores for you. To do this, select Column A Row 2, and type in =randbetween(1,100). A number between 1 and 100 should appear. Hover the mouse at the bottom right of the cell and you will see a +. Select and pull down to proliferate the column, which is Class 1 in the example below.
4. Do this for each class. You should end up with something that looks like this. It's up to you how many rows you fill, but I would suggest about 25.
   

   Class 1	Class 2	Class 3	Class 4	Class 5
   25	73	34	50	55
   86	14	57	16	19
   17	55	68	13	54
   43	87	90	90	49
   41	88	44	95	60
   1	38	43	55	40
   16	51	41	88	81
   24	61	78	11	91
   34	41	69	90	39
   66	99	24	52	77
   51	81	6	70	88
   24	85	52	54	76
   75	56	17	64	61
   25	80	71	71	93
   97	89	39	67	7
   7	45	25	64	42

5. Set up a table, such as the table above, to organise the summary statistics for each class and then calculate the mean, median, mode and range for each class.
6. What do you notice? Remember the real-world context here! If you were the lead mathematics teacher for this year level, what would you think? What would you want to say at the next mathematics meeting with all class teachers?
7. Let’s do further analysis by visualising this data on box plots. Select all the data and click the Insert tab.
8. Expand the Charts tab, scroll down all the different types of displays and select 'box plots'. In Excel, this is called 'Box and Whisker'. This action will insert side-by-side box plots. It should look something like this. 
   
9. Revisit your comments from earlier. What can you add? What needs to be adjusted?
10. Now imagine you have a meeting with the head of mathematics and all the mathematics teachers; prepare a short presentation for this next meeting to reflect on the outcomes of this test.

So, is teaching for you? There’s more to it than you think!

Success criteria

• I can use technology to generate datasets and display them effectively with side-by-side box plots.
• I can make accurate comparisons of datasets, referencing the centre, spread, shape and outliers and present it in an informative manner.