Planning tool
Year levels
Strands
Expected level of development
Australian Curriculum Mathematics V9: AC9M3N06
Numeracy Progression: Counting processes: P6, Additive strategies: P8, Number patterns and algebraic thinking: P4, Understanding money: P4
At this level, students use mathematical modelling to solve practical problems involving additive and multiplicative situations including financial contexts. Students apply their mathematical knowledge to formulate number sentences and choose relevant calculation strategies to come up with a solution. They communicate that solution referring to the problem context.
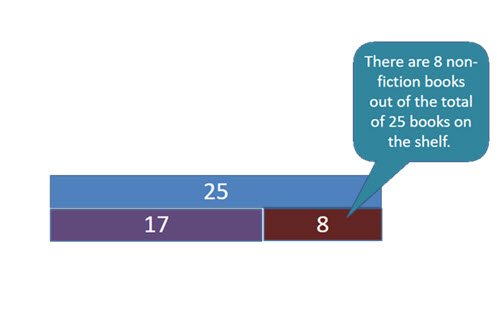
Present word problems and demonstrate using worked examples how a bar model can be used to represent the problem. Talk through the problem and what each part of the bar model relates to in connection with the problem. Explain how the structure of the bar model visually represents the relationships in the problem.
Use contexts that require multiplicative thinking to show how repeated addition or multiplication can be used to solve problems.
This topic has connections to developing efficient strategies for addition and subtraction calculations (AC9M3N03) as well as for calculations involving multiplication and division (AC9M3N04).
Teaching and learning summary:
- Present students with problems that encourage them to select the appropriate operation and apply known calculation strategies.
- Provide guidance on using mathematical modelling to solve number problems.
- Incorporate the routine of checking whether their mathematical model makes sense and ensure the answer is communicated in relation to the problem's context.
Students:
- identify and describe real-world problems involving additive or multiplicative relationships
- represent additive or multiplicative real-world problems mathematically as number sentences and using bar models
- choose and apply appropriate calculation strategies
- use a mathematical modelling process to solve practical, real-world problems involving additive or multiplicative strategies.
Some students may:
- need direction and guidance when solving a problem, as they may not yet feel confident in choosing an appropriate calculation strategy.
- require support in formulating problems using appropriate number sentences to represent the situation.
- not yet realise that the solution must be connected back to the context of the original problem, and may simply state an answer, for example, ‘five’.
To address these misconceptions:
- encourage students to identify key information in the problem and use this to create a number sentence that reflects the mathematical operations needed to solve it. For example, ‘If each carton contains 24 cans and there are 5 cartons, the number sentence would be 5 × 24 = 120.’
- prompt students to clarify their answers. For example, when a student says, ‘The answer is five,’ ask them to explain, ‘Five what?’ This helps them understand that the number alone is not the solution, but the answer needs to be framed in the problem's context.
- explicitly teach that solutions should connect back to the scenario. For example, in the problem, ‘Work out the number of cartons of soft drink cans needed for the entire Year 3 cohort,’ a more complete answer would be: ‘They would need five cartons of 24 cans each to ensure that every Year 3 student gets at least one can.’
The Learning from home activities are designed to be used flexibly by teachers, parents and carers, as well as the students themselves. They can be used in a number of ways including to consolidate and extend learning done at school or for home schooling.
Learning intention
- We are learning to use additive and multiplicative thinking to solve maths-based problems.
Why are we learning about this?
We use additive and multiplicative thinking everyday to solve problems and do mental calculations in various situations.
What to do
Complete these three tasks.
![]()
1.Setting the table
- Task: Draw your dining table set-up for a family dinner. Make sure to include a plate and three utensils (fork, knife and spoon) for each person.
- Question: How many plates and utensils are there altogether, for everyone at the table?
- Challenge: Write a number sentence to show your calculations.
![]()
2. Laundry
There are 20 socks in the pile. If there are 5 people in the family, how many pairs of socks will each person get?
- Show how you worked out the problem.
- What is your number sentence?
![]()
3. Movie tickets
Your parents have budgeted $50 for you and two friends to go to movies at the local cinema. Each ticket costs $15. Will you have enough money left for popcorn, which costs $2.50 a box?
- Show how you worked out the problem.
- What calculation strategies did you use?
Success criteria
I can:
- represent everyday maths problems mathematically as number sentences
- choose and apply appropriate calculation strategies to find solutions to the problems
- use visual representations to illustrate my thinking and support my calculations.
Please note: This site contains links to websites not controlled by the Australian Government or ESA. More information here.
Teaching strategies
A collection of evidence-based teaching strategies applicable to this topic. Note we have not included an exhaustive list and acknowledge that some strategies such as differentiation apply to all topics. The selected teaching strategies are suggested as particularly relevant, however you may decide to include other strategies as well.
-

Concrete, Representational, Abstract (CRA model)
The CRA model is a three-phased approach where students move from concrete or virtual manipulatives, to making visual representations and on to using symbolic notation.
Go to resource -
Classroom talks
Classroom talks enable students to develop language, build mathematical thinking skills and create mathematical meaning through collaborative conversations.
Go to resource -

Questioning
A culture of questioning should be encouraged and students should be comfortable to ask for clarification when they do not understand.
Go to resource
Teaching resources
A range of resources to support you to build your student's understanding of these concepts, their skills and procedures. The resources incorporate a variety of teaching strategies.
-

Additive strategies: Video and teaching guide
Use this video to explore the use of computation strategies, rounding and estimation in real-world, additive situations.
Go to resource -

Mathematical modelling: Word problems
This lesson introduces students to using mathematical modelling to solve practical problems involving both additive and multiplicative relationships.
Go to resource -

Using bar models to solve word problems (Part 1)
In this lesson, students solve word problems using bar models as a visual tool to aid comparison between two values.
Go to resource -

Using bar models to solve word problems (Part 2)
In this lesson, students solve word problems using bar models as a visual tool to aid addition and money problems involving change.
Go to resource -
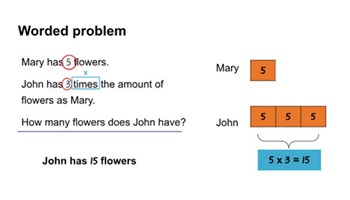
Using bar models to solve multiplication word problems
In this lesson, students learn to use a part-part-whole bar model to solve word problems that involve multiplication.
Go to resource -
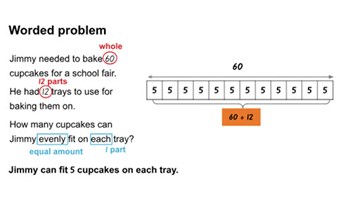
Using bar models to solve division word problems
In this lesson, students learn to use a part-part-whole bar model to solve word problems that involve division.
Go to resource
