Expected level of development
Australian Curriculum Mathematics V9: AC9M4N04
Numeracy Progression: Interpreting fractions: P5
At this level, students extend their understanding of the order of numbers locating fractions as numbers along a number line and counting by fractions.
Connect students’ use of an area or region model to visually represent common fractions such as thirds, quarters and halves on a number line to demonstrate the link between fractional parts and linear models. A think board can be used to build understanding and connections between the multiple representations of a fraction including visual models, equivalence and a relevant mathematical story.
Count fractions to develop fluency of fraction number names to and beyond the whole using a number line. For example, count by thirds and mark each fraction along a number line: one-third, two-thirds, three-thirds (one whole), four-thirds (one and one-third). Discuss converting improper fractions as a mixed number, such as 43 as 113 .
Use multiple examples to show how mixed numbers can be converted into improper fractions and represented on number lines. Enable students to observe the relationship between, for example, six-fifths and one and one-fifth (a concept that will be revisited when demonstrating the concepts of operations with fractions and decimal fractions).
Teaching and learning summary:
- Use number lines to show the link between region/area models and linear/line models of fractions.
- Utilise horizontal and vertical number lines in real-life contexts.
- Convert mixed numbers to improper fractions and model on number lines.
- Use correct language when discussing fractions (3 quarters, 3 parts of 4, not 3 over four).
The Learning from home activities are designed to be used flexibly by teachers, parents and carers, as well as the students themselves. They can be used in a number of ways including to consolidate and extend learning done at school or for home schooling.
Learning intention:
- We are learning to represent and count fractions.
Why are we learning about this?
- To show our understanding of fractions as a number along a number line.
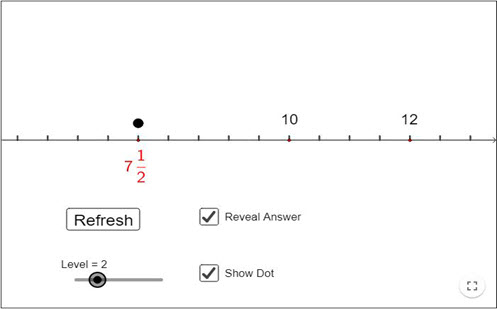
Before you begin, you can use the Fraction Number Line to explore the different fractions to refresh your thinking.
What to do
1. Look at these fractions and then decide if they are closer to 0, 1 or 2.
| 18 | 35 | 1 34 | 49 | 56 | 210 | 13 | 0.7 |
a. Place each of the fractions on a number line.
b. Which fraction is closest to:
0:
1:
2:
c. How do you know?
Success criteria:
I can:
- locate a fraction on a number line
- explain whether a fraction is closer to 0, 1 or 2.
Please note: This site contains links to websites not controlled by the Australian Government or ESA. More information here.
Teaching strategies
A collection of evidence-based teaching strategies applicable to this topic. Note we have not included an exhaustive list and acknowledge that some strategies such as differentiation apply to all topics. The selected teaching strategies are suggested as particularly relevant, however you may decide to include other strategies as well.
-
Explicit teaching
Explicit teaching is about making the learning intentions and success criteria clear, with the teacher using examples and working though problems, setting relevant learning tasks and checking student understanding and providing feedback.
Go to resource -
Questioning
A culture of questioning should be encouraged and students should be comfortable to ask for clarification when they do not understand.
Go to resource -
Classroom talks
Classroom talks enable students to develop language, build mathematical thinking skills and create mathematical meaning through collaborative conversations.
Go to resource -
Multiple exposures
Providing students with multiple opportunities within different contexts to practise skills and apply concepts allows them to consolidate and deepen their understanding.
Go to resource
Teaching resources
A range of resources to support you to build your student's understanding of these concepts, their skills and procedures. The resources incorporate a variety of teaching strategies.
-
Fruit fractions: Fruit salad crafty creations
Students explore fractions through creating their very own simulated ‘fruit salad’.
Go to resource -
Measuring sticks: Decimals
In this lesson, students learn about place value and how it extends beyond whole numbers.
Go to resource -
Number lines in disguise
Use this lesson with interactive tool to explore numbers represented on a number line including fractions.
Go to resource -
GeoGebra: Number lines in disguise
Dynamic software to explore numbers represented on a number line including fractions.
Go to resource -
Equivalent fractions
In this unit, students are introduced to exploring ways to find equivalent fractions including placing them on number lines.
Go to resource -
Comparing fractions using a number line
In this lesson, students compare fractions with different denominators by using number lines.
Go to resource
