Planning tool
Year levels
Strands
Expected level of development
Australian Curriculum Mathematics V9: AC9M4N02
Numeracy Progression: Multiplicative strategies: P6
At this level, students investigate the properties of odd and even numbers. They learn to identify and explain patterns that emerge when odd and even numbers are used in computation problems.
Provide multiple opportunities to identify numbers as odd and even. Use representations to help visualise properties of odd and even. For instance, use arrays to help students see that even numbers can be made into two equal row arrays, but odd numbers cannot. Link this to the idea that some collections can be shared evenly into two groups, without leaving a remainder, and some cannot.
Sort and classify numbers as odd or even and have students explain the significance of the ending digit of a number. Challenge students to classify small and large numbers as odd or even. Use interactive digital tools to explore properties of odd and even numbers.
Challenge students to make observations and generalisations about patterns that emerge when odd and even numbers are used in computation problems. For instance, they may have noticed that 2 + 2 = 4, 2 + 4 = 6, 2 + 6 = 8 and so make the claim that even + even = even. Ask student to hypothesise, test and record their discoveries under one of the following headings: Always True, Sometimes True, Never True. Invite students to gather evidence to support or disprove a claim.
Connect prior knowledge of repeating patterns to the study of odd and even numbers. For instance, use a hundreds chart to create visual patterns by colouring in even numbers in one colour and odd numbers in another. Help students see that ‘odd-even’ is a repeating unit in the whole number system and that 0 through to 9 are repeated in the numeral system.
Use multiplicative thinking strategies when exploring divisibility of numbers to decide whether a number is odd or even if it divisible by 2 without a remainder.
Introduce algorithms as a way to describe a series of steps to achieve an outcome. Use differentiated teaching strategies for students to either follow or create an algorithm to decide whether a number is odd or even.
Teaching and learning summary:
- Investigate odd and even numbers using multiple contexts and approaches.
- Prompt student reasoning about whether a number is odd or even.
- Introduce an algorithm to identify an odd or even number.
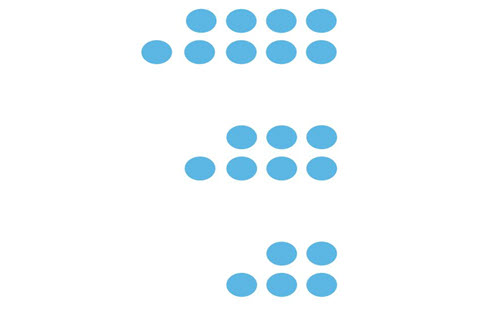
Students:
- explain that all numbers that end in 0, 2, 4, 6 and 8 are even, and that numbers ending in 1, 3, 5, 7 and 9 are odd
- recognise zero as a number that represents even properties
- explain why even numbers are divisible by 2 and why odd numbers are not divisible by 2
- explain why patterns involving odd and even are always true, for example, even + even = even, odd + odd = even, odd + even = odd.
Some students may:
- be confused by the function and properties of 0. Students may wonder whether it is odd, even or neither. Help students scrutinise zero based on the definition of an even number: Any number that can be divided by two to create another whole number. Half of zero equals zero so it fits this description. It also has odd numbers on either side of it (1 and –1), which further supports the case for zero being an even number.
- not appreciate the last digit of a whole number will determine whether the number is odd or even. Difficulties are compounded when zero is involved, for example, 100, 110 and 101. Give students opportunities to make or draw physical collections of these numbers. Ask students to recall the properties of even numbers – numbers with a remainder of zero when divided by 2. A 1–120 number chart can help students investigate the function of zero in numbers beyond 100 and help them recognise the continuation of the odd/even pattern.
- be unable to identify even numbers when the number includes odd digits. For example, they may classify 38 as odd because of the 3 in the tens place. To address this, provide repeated opportunities to visualise and recognise that multiples of 10 are always even, and that it is the number in the ones place that determines whether the whole number is odd or even.
- hold misconceptions about the rules that make a number even or odd. For example, they may think that when two odd numbers are added the sum will be odd. To address this, provide repeated opportunities to explore and notice how applying operations to numbers makes them odd or even.
The Learning from home activities are designed to be used flexibly by teachers, parents and carers, as well as the students themselves. They can be used in a number of ways including to consolidate and extend learning done at school or for home schooling.
Learning intention
- We are learning what makes numbers odd and even.
Why are we learning about this?
- Understanding odd and even numbers and their properties supports our capacity and confidence for solving number problems.
What to do
- Go for a local walk with someone in your family. Notice how house numbers don’t ‘count’ in the usual way. Usually, one side of the street has even-numbered houses (numbers ending in 0, 2, 4, 6, 8) and the other has odd-numbered houses (numbers ending in 1, 3, 5, 7, 9). Once the pattern is understood, ask your child: ‘What will the next house number be?’ Discuss the pattern that skip-counts by two.
- Keep a hundreds chart on the fridge and look for patterns to do with odd and even numbers.
- Come up with a rhyme or song to remember which numbers are odd and which are even.
- Ask questions such as: ‘What do you know about odd/even numbers?’ ‘What are all the even/odd numbers between zero and twenty?’
- Notice and explore patterns involving odd and even numbers. For instance, you might notice that 1 + 1 = 2, 3 + 3 = 6 and 5 + 5 = 10 and so make the claim that odd + odd = even. Gather more evidence to support or disprove this claim.
Success criteria
I can:
- skip count by twos to count objects
- identify whether a number is odd or even
- explain what makes a number odd or even
- explain why patterns such as even + even = even (for example, 4 + 6 = 10) and even – even = even (for example, 10 – 6 = 4) are true.
Please note: This site contains links to websites not controlled by the Australian Government or ESA. More information here.
Teaching strategies
A collection of evidence-based teaching strategies applicable to this topic. Note we have not included an exhaustive list and acknowledge that some strategies such as differentiation apply to all topics. The selected teaching strategies are suggested as particularly relevant, however you may decide to include other strategies as well.
-

Collaborative learning
For group work to be effective students need to be taught explicitly how to work together in different settings, such as pairs or larger groups, and they need to practise these skills.
Go to resource -

Concrete, Representational, Abstract (CRA model)
The CRA model is a three-phased approach where students move from concrete or virtual manipulatives, to making visual representations and on to using symbolic notation.
Go to resource -

Differentiated teaching
Differentiation involves teachers creating lessons that are accessible and challenging for all students.
Go to resource -

Multiple exposures
Providing students with multiple opportunities within different contexts to practise skills and apply concepts allows them to consolidate and deepen their understanding.
Go to resource -

Questioning
A culture of questioning should be encouraged and students should be comfortable to ask for clarification when they do not understand.
Go to resource
Teaching resources
A range of resources to support you to build your student's understanding of these concepts, their skills and procedures. The resources incorporate a variety of teaching strategies.
-

It is odd – or is it?
In this lesson, students investigate the properties of odd and even numbers.
Go to resource -
resolve: Algebra: odds and evens
In these two lessons, students develop their understanding of the properties of odd and even numbers by exploring the results of adding and subtracting odd and even numbers.
Go to resource -

Largest even
This interactive tool enables students to explore even numbers and develop an understanding of odd and even numbers.
Go to resource -

Always, sometimes or never? Number
Use this task to explore odd and even numbers, make predictions and explain findings using reasoning.
Go to resource -

Play to 37
Use this game as a rich investigation into the adding together of odd numbers.
Go to resource -

An odd spell of maths
Use this lesson to investigate adding odd and even numbers and find solutions for addition patterns.
Go to resource -
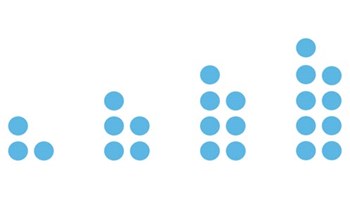
A dotty talk
Use this visual prompt to elicit observations about odd and even numbers.
Go to resource -

Make it even
Use this open-ended task to investigate the conditions that make a number odd or even.
Go to resource -
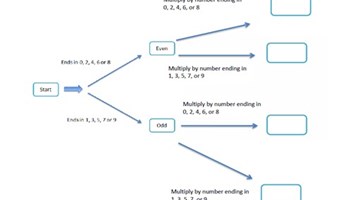
Odd or even machine
Use this lesson to incorporate algorithmic thinking and properties of odd and even numbers.
Go to resource -
That's odd
This is a level 2 and 3 algebra link activity from the Figure It Out series. It is focused on finding patterns for adding and multiplying odd and even numbers. A PDF of the student activity is included.
Go to resource
