Planning tool
Year levels
Strands
Expected level of development
Australian Curriculum Mathematics V9: AC9M4SP03
Numeracy Progression: Understanding geometric properties: P4
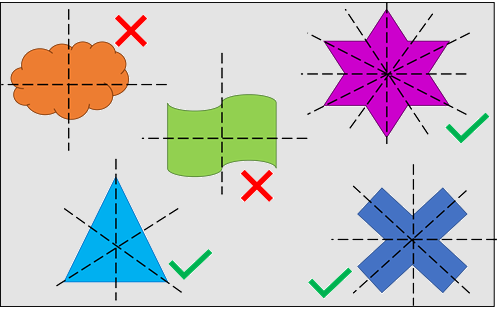
At this level, students investigate reflection symmetry and develop their understanding of how the line of symmetry defines the two aspects of a ‘mirror’ image of the figure into two congruent halves. They explore rotational symmetry using familiar 2D shapes, letters or numbers.
Make explicit through relevant examples, modelling and investigation that a figure can have one line of symmetry, several lines of symmetry, or no lines of symmetry.
Teaching and learning summary:
- Model how to work out if a figure has a line of symmetry.
- Use practical activities to explore symmetry.
- Look for examples of symmetry in nature and other real world objects.
Students:
- draw missing portions of a symmetrical figure
- describe the line and rotational symmetry by manually cutting, folding and turning shapes and by using digital technologies
- explain why some figures have no line of symmetry.
Some students may:
- have trouble visualising the line of symmetry and mistakenly draw a line of reflection along an incorrect axis. Use practical examples where students fold simple shapes to check their predictions.
- identify one line of symmetry and not understand a shape may have more than one line of symmetry. Use a task which challenges students to find as many lines of symmetry as possible or provide shapes that have multiple lines of symmetry and make explicit the lines of symmetry.
- believe that, like a square, a rectangle has 4 lines of symmetry when it only has two. Use practical examples where students fold a rectangle to check their predictions.
The Learning from home activities are designed to be used flexibly by teachers, parents and carers, as well as the students themselves. They can be used in a number of ways including to consolidate and extend learning done at school or for home schooling.
Learning intention
- We are learning about symmetrical shapes.
Why are we learning about this?
- Symmetry is all around us. You can find examples in nature, art, building and design.
What to do
- Have a look at this image. What is it?
- Can you draw the half that is missing?
- How do you know what to draw?
- Explain your answer.

- Complete these pictures.
- If you have a mirror, reflect the image and draw what you see.




- If you have a mirror, reflect the image and draw what you see.
- Would this activity work for all objects? Why or why not?
Success criteria
I can:
- complete the remaining half of an object that is symmetrical
- describe what symmetry is and what it isn’t with some examples.
Learning intention
- We are learning how to define symmetry.
- We are learning how to draw shapes or objects with their lines of symmetry.
Why are we learning about this?
- We are using symmetry to recognise and form patterns.
What I want you to do
- Here are two letters of the alphabet.
- Which one is symmetrical?
- Which one has no line of symmetry?
- How did you work it out?
A
G
- Look at the 26 letters of the English alphabet.
- Write each one in capital letters.
- Estimate how many of the letters are symmetrical.
- Check to see if each letter has a vertical line of symmetry or a horizontal line of symmetry or both.
- Draw your results to show how you worked out which letters are symmetrical. How did your estimate compare?
Success criteria
I can:
- recognise when a letter has no lines of symmetry
- identify the lines of symmetry.
Please note: This site contains links to websites not controlled by the Australian Government or ESA. More information here.
Teaching strategies
A collection of evidence-based teaching strategies applicable to this topic. Note we have not included an exhaustive list and acknowledge that some strategies such as differentiation apply to all topics. The selected teaching strategies are suggested as particularly relevant, however you may decide to include other strategies as well.
-

Mathematics investigation
By giving students meaningful problems to solve they are engaged and can apply their learning, thereby deepening their understanding.
Go to resource -

Explicit teaching
Explicit teaching is about making the learning intentions and success criteria clear, with the teacher using examples and working though problems, setting relevant learning tasks and checking student understanding and providing feedback.
Go to resource -

Culturally responsive pedagogies
Culturally responsive pedagogy is a form of teaching that incorporates learners’ cultural background and histories into classroom practice.
Go to resource
Teaching resources
A range of resources to support you to build your student's understanding of these concepts, their skills and procedures. The resources incorporate a variety of teaching strategies.
-

Symmetry hunt
Explore line and rotational symmetry using suggested learning tasks.
Go to resource -

Line of symmetry
Students identify and draw the lines of symmetry by folding 2D shapes.
Go to resource -

Fold and cut
In this unit, students explore line or reflective symmetry. Students develop an understanding of what line symmetry is and the names and attributes of common 2D mathematical shapes.
Go to resource -

Colouring triangles
Students explore ways of colouring a set of triangles so that they make symmetrical patterns.
Go to resource -

reSolve: Authentic problems – expanded square
This sequence of four lessons explores concepts around informal area and symmetry.
Go to resource -

reSolve: Transformations – frieze patterns
This sequence of three lessons explores transformation and symmetry by engaging students in the design of friezes.
Go to resource -

Making maths: snowflakes
This task shows students how to fold and cut paper to make a model snowflake, demonstrating six lines of symmetry.
Go to resource -

Getting in line
A useful starting point to explore line symmetry in 2D shapes.
Go to resource -

Pattern matching
In this unit, students explore lines of symmetry in pictures, shapes and patterns and use their own words to describe the symmetry.
Go to resource -

Sea symmetry
Use a mirror to explore line (reflection) symmetry to complete a drawing.
Go to resource -

Grid symmetry
Use a problem-solving approach to investigate reflection symmetry.
Go to resource -

Finding lines of symmetry
Students explore line symmetry by drawing lines of symmetry to divide shapes into two halves.
Go to resource -

Geometric pictures of one half
Students draw on geometric reasoning, symmetry and understanding of a half to compare the area of a square.
Go to resource -
Fold and cut 2
This unit involves folding and cutting paper to produce and perceive number patterns and geometric shapes.
Go to resource
