Planning tool

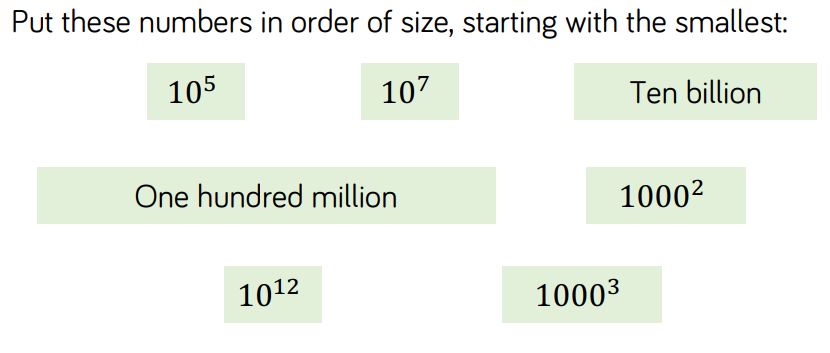
Students:

• use exponent notation to expand and contract numbers
• convert between natural numbers in expanded form and powers of 10
• are fluent with place value
• sequence powers of 10 for big and small numbers.

Some students may:

• often make the mistake of multiplying the base by the index instead of multiplying the base by itself for the number of times represented by the index. Students who make this error will say, for example, 3²= 6 (instead of 9).
• incorrectly think that multiplying by an index number of zero will give the answer zero.
• get confused between having a negative on the index number and a negative on the base.

The Learning from home activities are designed to be used flexibly by teachers, parents and carers, as well as the students themselves. They can be used in a number of ways including to consolidate and extend learning done at school or for home schooling.

Learning Intention

• I am learning about positive indices, negative indices and zero indices.
• I am learning that exponents are used to write repeated multiplication.

Why are we learning about this?

Exponential notation is an effective way to write what could be a longer complicated multiplication problem.

What to do

1. Go to the number slider and print it out.
2. Find some scissors and first cut out the large orange box and then cut out the white box below it.
3. Fold the orange box along the dotted line backwards so that the white boxes are facing you.
4. Cut out the nine white boxes to make small windows underneath the words that say Ten-thousands, Thousands, Hundreds, Tens etc.
5. Fold the orange box backwards so the windows are facing up.
6. Cut out the white rectangular piece that was below the orange box, and then slide it into the slider.

To the left

1. Pick two numbers and write one of them in the Ones box and the other in Tenths box. I’ve chosen 1 and 0.
2. What happens when you pull your white strip from the left? I get 10. What about you? What did you get?
3. Slide your numbers to the left for a second time. Hmm … I better add another zero to make it a proper number around the decimal point. Mine becomes 100. What about your number?
4. Keep pulling from the left and adding zeroes, or any number you want. What is happening to your number?
5. Is it getting bigger of smaller?

To the right

1. Return the numbers back to their original positions.
2. What happens to your numbers when you slide the strip to the right? Mine became .10 What happens when you slide the strip two positions to the right, and then three positions and then four? What do you think is happening to your number?
3. Is it getting bigger of smaller?
4. What operation are you using time when you slide your number to the left?
5. What operation are you using when you slide your number to the right?

Success criteria

• I can understand the significance of exponents to shorten big numbers or small numbers.
• I can work with both positive and negative exponents on a number line.