Expected level of development
Australian Curriculum Mathematics V9: AC9M8N02
Numeracy Progression: n/a
At this level, students extend the exponent laws of numerical calculations involving positive integers and zero exponents, and solve a broad range of practical problems, using mental methods, written algorithms and digital tools.
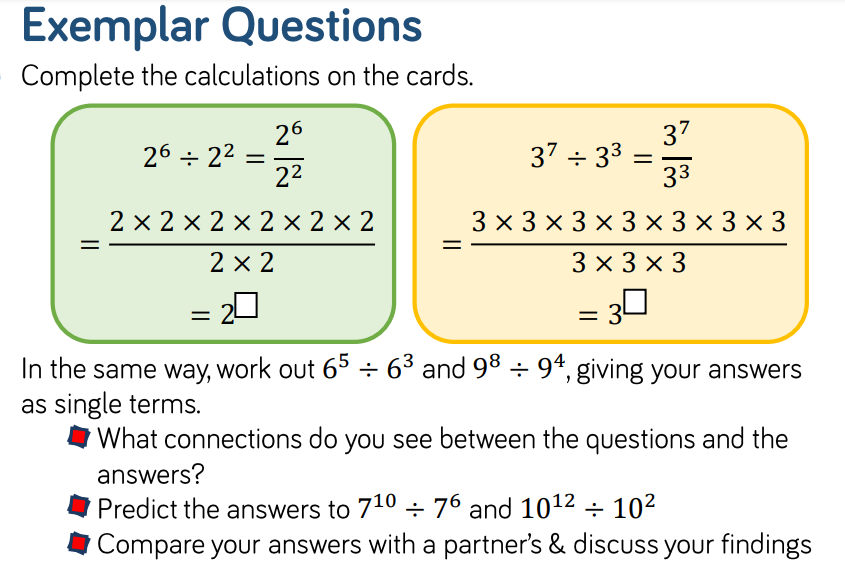
Discuss that the terms 'power', 'index' (indices) and 'exponent' have the same meaning. Explain why we write numbers in this way and how it is useful in calculations. It’s important to visually show the expanded form and simplifying an expression, and the different ways it can be represented. For example,
33 × 35 = (3 × 3 × 3) × (3 × 3 × 3 × 3 × 3) = 38 where 3 is the base number. Students also need explicit instruction for the location of the base and the exponent. Draw a link between the word 'base' and its location.
Introduce the product of powers rule, quotient of powers rule and the powers of power rule. Allow students to explore the exponent laws themselves by writing numbers in expanded form. Deriving the laws for themselves will deepen the their understanding. Allow students to find the patterns for expanded and simplified expressions but demonstrate how we multiply, divide and subtract exponents with calculations. This will be challenging for some, and a summary can be provided to the class to ensure they all have a solid understanding. Use this opportunity to model how summary notes should be laid out.
Demonstrate the zero-power rule using the quotient of powers rule. Demonstrate what happens when you divide a number itself, and what happens when you subtract a number by itself. When applying this to exponents, what does this tell us?
Go deeper and apply these laws to problems that can be solved in two steps and consolidate using examples from other areas. Use digital tools to help illustrate the applications of exponents and how they can be solved. Provide students with examples of problems that combine the laws so they can practise simplifying complex expressions.
Teaching and learning summary:
- Explore positive integer exponents and show how they can be written in expanded form.
- Apply exponent laws to positive integers and demonstrate the zero-exponent law.
- Solve problems using exponent laws and use digital tools for interactivity.
- Allow students to derive the index laws for themselves.
- Teach students the vocabulary of exponent notation, and that there are many words that might be used for the same term.
Please note: This site contains links to websites not controlled by the Australian Government or ESA. More information here.
Teaching strategies
A collection of evidence-based teaching strategies applicable to this topic. Note we have not included an exhaustive list and acknowledge that some strategies such as differentiation apply to all topics. The selected teaching strategies are suggested as particularly relevant, however you may decide to include other strategies as well.
-
Explicit teaching
Explicit teaching is about making the learning intentions and success criteria clear, with the teacher using examples and working though problems, setting relevant learning tasks and checking student understanding and providing feedback.
Go to resource -
Feedback
It has been shown that good feedback can make a significant difference to a student’s future performance.
Go to resource -
Questioning
A culture of questioning should be encouraged and students should be comfortable to ask for clarification when they do not understand.
Go to resource
Teaching resources
A range of resources to support you to build your student's understanding of these concepts, their skills and procedures. The resources incorporate a variety of teaching strategies.
-
Applying the properties of exponents
This lesson unit will help teachers to assess students' understanding and application of indices. There are lesson plans and exercises, and advice on assessment.
Go to resource -
Multiples, factors and powers
This teacher guide covers in detail exponent laws and using exponent notation. Many examples and questions are provided.
Go to resource -
Putting Life on Mars
Students develop fluency by using knowledge about place value to make sense of very large and very small numbers.
Go to resource
Assessment
By the end of Year 8, students can apply the exponent laws to calculations with numbers involving positive integers exponents.
-
Exponents and scientific notation
Set students numerous assessments on the topic of exponents using this resource. Teacher guidance and explanations are also given for scaffolding purposes.
Go to resource -
MYMC Level 8: Proficiency Rubrics
A formative assessment rubric template for the Level 8 Middle Years Maths Challenges that teachers can modify and tailor to each challenge and proficiency.
Go to resource