Possible outcomes : Year 8: Planning tool

Expected level of development

Australian Curriculum Mathematics V9: AC9M8P01

Numeracy Progression: Understanding chance: P6

At this level, students are introduced to more complex probability concepts, terminology and visual representations. These concepts may be challenging for students to comprehend and will need multiple examples incorporating relevant teaching strategies.

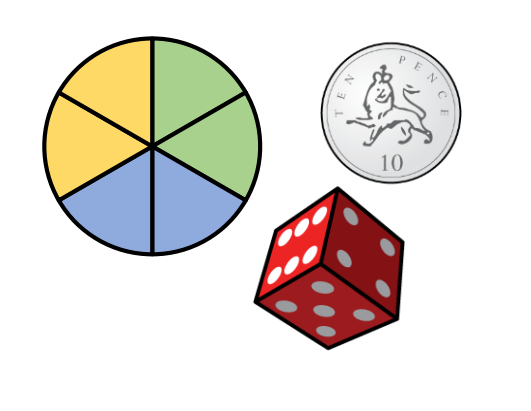
A complementary event occurs when there are only two outcomes. In this situation the probability sum of these events must be 1. Students can then calculate the probability of an event not occurring. The use of concrete materials and hands-on activities helps students come to a deeper understanding of this concept; for example, rolling a number higher than 4 with a dice, is a 2 in 6 chance, which means that there is a 4 in 6 chance that a number higher than 4 won’t be rolled in a series of trials. Demonstrate this with a large number of trials using tables to record results.

From here, make connections with numerical calculations.

Recall that proportions are ratios and ratios are fractions and that we can use fractions to make calculations. Use probability notation to help with fluency.

For a single event (A), the Pr(A) + Pr(not A) = 1.

Then represent the thinking as fractions. Using our example:

since 26 + 46 = 1; therefore, 1 – 26 = 46

When students are comfortable with this concept, provide various opportunities for them to derive this relationship from worded problems and contexts.

Discuss chance data reported in the media and apply students' understanding of probability to explain meaning and the accuracy of data.

Teaching and learning summary:

Revise previous knowledge of probability.
Define the terms clearly and use worked examples to make the differences between the terms clear.
Introduce the concept of complementary events – their probabilities add to 1.
Encourage students to use the language and notation of probability.

Students will:

be able to identify complementary events
derive meaning from a variety of contexts and make calculations for single events
know and be able to use the language of probability accurately.

Some students may:

believe that when there are two possibilities, they must be equally likely (perhaps because so many classroom and textbook examples use coin tossing).
confuse the difference between experimental and theoretical probability.

Encourage students to read questions carefully as slight nuances in language are important in probability. Students whose first language is a language or dialect other than English may need particular support in this topic.

The Learning from home activities are designed to be used flexibly by teachers, parents and carers, as well as the students themselves. They can be used in a number of ways including to consolidate and extend learning done at school or for home schooling.

Learning intention

We are learning the concept behind complementary events.
We are learning to use household tools and games to discover and explore probability.

Why are we learning about this?

Probability is used in the world every day. Meteorologists, for instance, use probability to predict the weather. To learn about probability means that you can make sense of the world around you and understand how we make predictions and use numbers to create forecasts.

What to do

1. Find a pack of cards and make sure they are all there; there should be 52 cards of spades, hearts, diamonds and clubs. Gather a notebook and pen or open up a page on your device to take notes.

2. Shuffle the cards and place the pack in front of you.

3. Consider what the probability is of drawing a ‘10’ from the deck on your first try.

Trial number	What number did you draw?	Probability of drawing 10	Probability of not drawing 10	Probability of drawing any other card
1
2
3
4

5. Now try it! Pick a card and record your result on the first line in the table. Fill out all three columns.

6. Replace the card underneath and try again. Record your results.

7. Keep playing until you get a 10.

8. Was it easy? How many times did you have to try? Do you notice any patterns in the columns of your table? Do any of your probabilities (per row) add to 1?

Success criteria

I can use a simple game to help me understand probability and complementary events.
I understand that probability is all around me.

Please note: This site contains links to websites not controlled by the Australian Government or ESA. More information here.

Planning tool

Year levels

Strands

Topics