Formulate and manipulate expressions: Year 9: Planning tool

Expected level of development

Australian Curriculum Mathematics V9: AC9M9A02

Numeracy Progression: Number patterns and algebraic thinking: P9

At this level, students move beyond expanding and factorising linear expressions, such as 3(2x – 1) or 4x + 6, to working with monic quadratic expressions (that is, where the coefficient of the square term is 1). This involves expanding expressions of the form (x + a)(x + b), such as (x + 2)(x – 8), and factorising expressions of the form x² + mx + n, such as x² – 4x + 3.

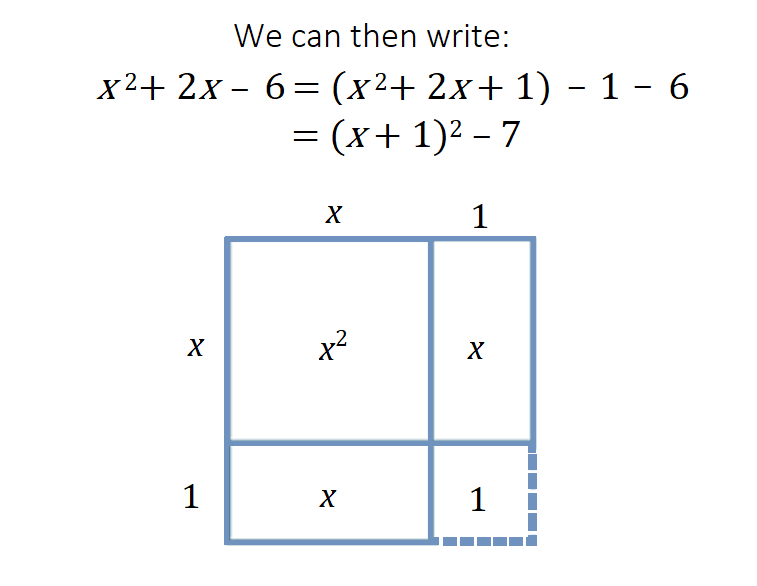
While students will have previously worked with squaring numbers and squaring in area calculations, this may be the first time they regularly come across the term ‘quadratic’ in reference to algebraic expressions. The abstract representation of these expressions, in expanded and factorised forms, may seem daunting. Small, step-by-step explicit instruction using concrete manipulatives (algebra tiles or area models) or digital tools is helpful for this topic.

It is important to consistently use the language and conventions of algebra when developing and demonstrating various concepts and processes, so that students progressively become more comfortable with formulating and manipulating quadratic expressions. They can then start to make connections from learning abstractly to systematically exploring and identifying patterns and relationships between expansion and factorisation based on
(x + a)(x + b) = x² + (a + b)x + ab, where a and b are integers.

By the end of this topic, students should be able to identify x², x and constant terms with integer coefficients, in expanded or factorised forms of monic quadratic expressions, and move between equivalent expanded and factorised representations.

Teaching and learning summary:

Use worked examples to show the combinations of binomials so that students can practise expansion skills.
Show the process for factorising monic quadratic expressions.
Use concrete manipulatives and digital tools to aid explanation.
Ensure students can expand (x + a)(x + b) and factorise
x² + mx + n , where m and n are integers.

Students:

are familiar and comfortable with the conventions and language of quadratic expressions
can formulate and manipulate a variety of linear and quadratic expressions
can expand binomial products
can factorise monic quadratic expressions
make connections between factorisation and expansion in relation to monic quadratic expressions.

Some students may:

not understand the convention of implied multiplication. This could mean that they still need to learn that 6x stands for 6 multiplied by x or 6 lots of x, or that ab stands for a × b.
be incorrectly calculating expressions involving negative integers, for example, thinking –2 × –3 = –6 rather than +6, or with simple algebraic operations such as the combination of like terms.
not correctly or completely apply the distributive property when expanding the product of two binomial expressions, such as simply missing out middle terms, incorrectly thinking (x + 3)² = x² + 9.

The Learning from home activities are designed to be used flexibly by teachers, parents and carers, as well as the students themselves. They can be used in a number of ways including to consolidate and extend learning done at school or for home schooling.

Learning intention

I am learning to recognise quadratic expressions and describe their terms.
I am learning to expand the product of two binomial expressions.
I am learning to factorise simple quadratic expressions.

Why are we learning about this?

Quadratic functions and their rules can be used to model situations and solve problems involving curves, area, patterns and movement. Formulating and manipulating quadratic expressions provides general ways to describe and understand these functions, expanding the range of practical problems we can solve.

What to do

Practise expanding

Use the interactive Quadratics - expand brackets – GeoGebra to practise expanding. Tick the ‘show working’ box and set the sliders for a and c to 1.
Use any method to expand each of the following:

(x + 3)(x + 5) (x + 3)(x – 5) (x – 3)(x + 5) (x – 3)(x – 5)
Repeat these combinations for several other pairs of numbers, and in each case compare the x term and the constant term in the expanded form with the two numbers in the factorised form.
Describe any patterns you notice.
See if you can use your pattern to expand the following in one step:

(x + 5)(x + 2) (x + 4)(x – 6) (x – 3)(x + 7) (x – 8)(x – 1)

Practise factorising

Use the interactive Quadratics factorisation game – GeoGebra to practise factorising (move the sliders to show what you think the answer is, and then check it using the 'check answer' button).

Success criteria

I can identify and describe the terms in a quadratic expression.
I can expand the product of two binomial expressions.
I can factorise monic quadratic expressions.

Please note: This site contains links to websites not controlled by the Australian Government or ESA. More information here.

Planning tool

Year levels

Strands

Topics